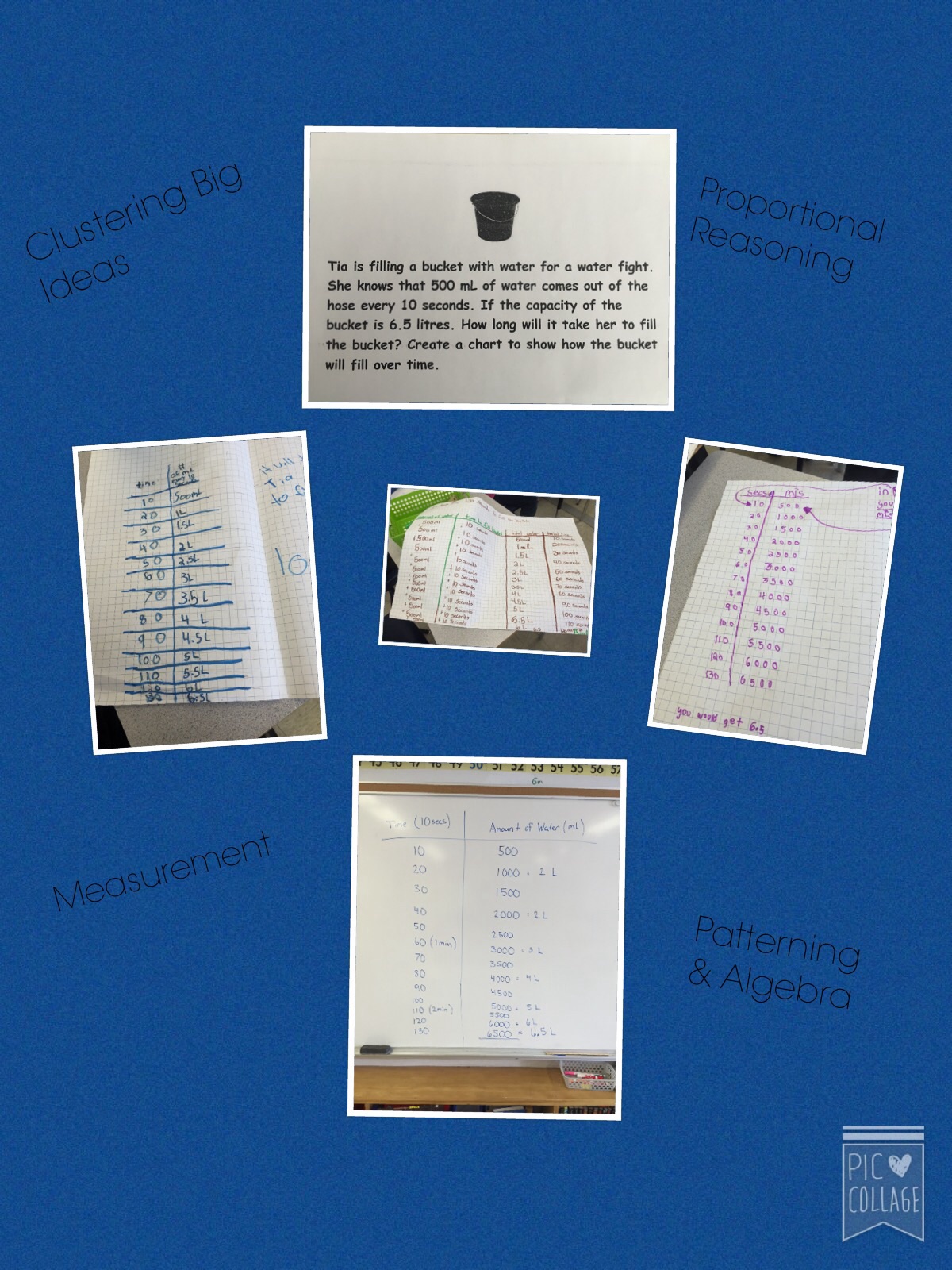
I love problems that provide authentic opportunities to cluster several big ideas. Recently, I was in a classroom where the teacher gave the following problem to his students.
Tia is filling a bucket with water. She knows that 500 ml of water comes out of the hose every 10 seconds. If the capacity of the bucket is 6.5 litres, how long will it take her to fill the bucket? Create a chart to show how the bucket will fill over time.
Big Idea # 1: Proportional Reasoning: A rate compares quantities with different units, for example, capacity to time. Once you know the rate for one comparison you can determine the values for different amounts (e.g., when I know I can fill a 5 litre bucket in 10 minutes then a 10 litre bucket will take 20 minutes.
Big Idea #2: Measurement: The unit chosen for a measurement affects the numerical value of the measurement. If you use a bigger unit, fewer units are required (e.g., 1 litre = 1000 ml)
Big Idea #3: Patterning & Algebra: Some ways of displaying data highlights patterns (e.g., t-chart).
Some students found it challenging to begin their charts so we asked the question: What are you being asked to find out? ( How long it would take to fill a 6.5 L bucket.)
What important information are we given? (In 10 seconds, 500 ml of water comes out of the hose.)
Highlighting this information helped students figure out what the headings should be for their chart. We continued to fill in the chart going up by 10 second increments each time. Students were able to see patterns across and down the chart. Through our questioning we moved students from saying that the pattern for the water was increasing each time by 500 ml to looking at different number relationships. They were observing that if we doubled the time then the water had to double as well. The chart provided the opportunity for us to see many doubling, tripling and halving opportunities (multiplicative thinking). Students were able to find out that it took 130 seconds or 2 minutes 10 seconds to fill the 6.5 L bucket, but we also wanted them to understand that they could now figure out any amount of water or time based on the given rate (proportional thinking). We asked questions like: How long would it take to fill 2 buckets? In 200 seconds how much water would come out of the hose? The chart helped highlight patterns and our questioning moved them to using number relationships to answer those questions (multiplicative thinking).